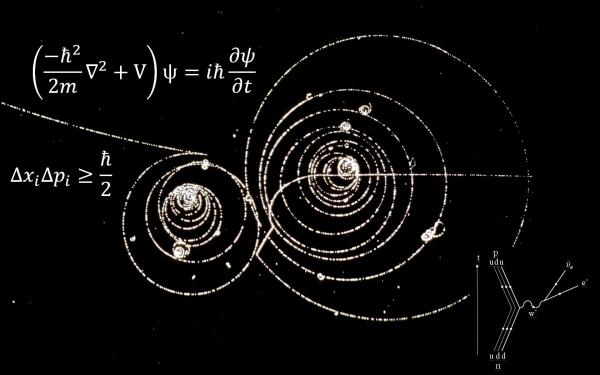
Bohr theory of atom

Bohr theory of atom is one of the initial achievement and application of quantum mechanics or old quantum mechanics as most would call it. It was able to explain the spectrum of hydrogen atom. Basic postulates of Bohr theory of atom are

a. An electron cannot revolve around the nucleus in all possible orbits as suggested by classical mechanics but the electron can revolve round the nucleus only in those allowed orbits for which the angular momentum of the electron is an integral multiple of $\hbar$ where $\hbar=\dfrac{h}{2\pi}$

b. An atom radiates energy only when an electron jumps from a stationary orbit of higher energy to one of lower energy. If the electron jumps from an initial orbit of energy $E_i$ to a final orbit of energy $E_f$ and $E_i > E_f$ then a photon with frequency $\nu = \dfrac{E_i-E_f}{h}$ is emitted.

Mathematical approach for Bohr theory of atom

Let us consider an atom with one electron of mass m moves with velocity $\nu$ in a circular orbit of radius $r$ centered at the nucleus. The charge of the electron is $-e$ and that of the nucleus is $+Ze$ . The centripetal force is equal to the coulomb force between electron and nucleus.

$\dfrac{m\nu^2}{r}=\dfrac{1}{4\pi\epsilon_0}\dfrac{Ze^2}{r^2}$ … (a)

The kinetic energy of the electron is

$T=\dfrac{1}{2}m\nu^2=\dfrac{1}{4\pi\epsilon_0}\dfrac{Ze^2}{2r}$ … (b)

According to Bohr, the stationary states of the system are characterized by definite values of the angular momentum such that,

$p_{\phi}=m \nu r = n\hbar$ n=1,2,3,……… ; … (c)

The velocity of the electron in its $n^{th}$ orbit is;

$\nu=\dfrac{n\hbar}{mr}$ … (d)

Let us put equation (d) in equation (b) and the radius of the orbit is obtained as;

$r_n=4\pi\epsilon_0 \dfrac{n^2\hbar^2}{mZe^2}$ … (e)

For the ground state of hydrogen atom n=1 and Z=1; the radius of the orbit is,

$r_0=4\pi\epsilon_0 \dfrac{\hbar^2}{me^2}= 0.528 A^0$

This quantity is known as Bohr radius.

The total energy of the atom is the sum of kinetic and potential energies.

$E=T+V=\dfrac{1}{4\pi\epsilon_0}(\dfrac{Ze^2}{2r}-\dfrac{Ze^2}{r})=-\dfrac{1}{4\pi\epsilon_0}\dfrac{Ze^2}{2r}=-T$

The energy of the system in its $n^{th}$ state is;

$E_n=-\dfrac{1}{8\pi\epsilon_0}\dfrac{Ze^2}{r} = -\dfrac{mZ^2e^4}{8\epsilon_0^2n^2h^2}$ …. (f)

As the value on n increases $E_n$ increases and the result is that the outer orbits have greater energies than the inner orbits.

Spectral series

If an electron jumps from higher energy orbit $n_i$ to lower energy orbit $n_f$ , the frequency of energy emitted is given by

$\nu=\dfrac{E_{n_i}-E_{n_f}}{h}$ .

Here using equation (f)

$E_{n_i}=-\dfrac{me^4}{8\epsilon_0^2h^2}\dfrac{1}{n_i^2}$
and

$E_{n_f}=-\dfrac{me^4}{8\epsilon_0^2h^2}\dfrac{1}{n_f^2}$
Therefore

$\nu=\dfrac{me^4}{8\epsilon_0^2h^3}[\dfrac{1}{n_f^2}-\dfrac{1}{n_i^2}]$

Wave number $\bar{\nu}$ of a radiation is defined as the reciprocal of its wavelength in vacuum;

$\bar{\nu}=\dfrac{1}{\lambda}=\dfrac{\nu}{c}$

$\bar{\nu}=\dfrac{me^4}{8\epsilon_0^2ch^3}[\dfrac{1}{n_f^2}-\dfrac{1}{n_i^2}]=R_H[\dfrac{1}{n_f^2}-\dfrac{1}{n_i^2}]$

Here

$R_H=\dfrac{me^4}{8\epsilon_0^2ch^3}=1.096 \times 10^7m^{-1}$

is the Rydberg constant.

Hydrogen spectrum is produced when the electron falls from outer orbit into innermost orbit. The five spectral series of hydrogen atom, named for their discoveries are
$n_f=1$ Lyman series (ultraviolet region)
$n_f=2$ Balmer series (visible region)
$n_f=3$ Paschen series (infrared region)
$n_f=4$ Brackett series (infrared region)
$n_f=5$ P fund series (infrared region)