Let P be a particle on a smooth plane inclined at an angle alpha to the horizon. If ABC represent a vertical section of the inclined plane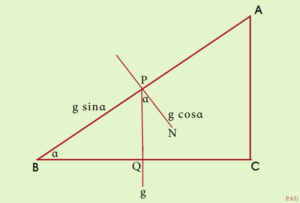motion down inclined plane through P, AB is the line of greatest slope and the angle ABC is alpha. The acceleration of P due to gravity is g and it is vertically downwards towards PQ. If PN be normal to the plane then angle QPN is alpha. The resolved parts of g are g cos\alpha along PN and g sin \alpha along PB.

Since the plane prevents any motion perpendicular to it, the particle moves down the plane along AB with acceleration g sin \alpha

If h be the height AC and l the length AB of the inclined plane sin \alpha = \dfrac{h}{l}

Let a particle slide down AB from rest and v be its velocity at B.

Then v^2 = 2gsin\alpha l = 2gh

Thus the velocity acquired is independent of alpha and is the same as the velocity acquired in falling through a height h.

If t be the time taken to slide down AB, l = \dfrac{1}{2}g sin\alpha t^2

t = \sqrt{\dfrac{2l}{g sin\alpha}}

If the particle is projected upwards along BA, its acceleration is -g sin\alpha